Reduced-Order Shape Optimization Using Offset Surfaces
Proceedings of the 2015 SIGGRAPH Conference
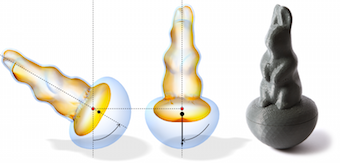
Given the 2-manifold surface of a 3d object, we propose a novel method for the computation of an offset surface with varying thickness such that the solid volume between the surface an its offset satisfies a set of prescribed constraints and at the same time minimizes a given objective functional. Since the constraints as well as the objective functional can easily be adjusted to specific application requirements, our method provides a flexible and powerful tool for shape optimization. We use manifold harmonics to derive a reduced-order formulation of the optimization problem which guarantees a smooth offset surface and speeds up the computation independently from the input mesh resolution without affecting the quality of the result. The constrained optimization problem can be solved in a numerically robust manner with commodity solvers. Furthermore, the method allows to simultaneously optimize an inner and an outer offset in order to increase the degrees of freedom. We demonstrate our method in a number of examples where we control the physical mass properties of rigid objects for the purpose of 3d printing.
@article{musialski-2015-souos,
title = "Reduced-Order Shape Optimization Using Offset Surfaces",
author = "Przemyslaw Musialski and Thomas Auzinger and Michael Birsak
and Michael Wimmer and Leif Kobbelt",
year = "2015",
abstract = "Given the 2-manifold surface of a 3d object, we propose a
novel method for the computation of an offset surface with
varying thickness such that the solid volume between the
surface an its offset satisfies a set of prescribed
constraints and at the same time minimizes a given objective
functional. Since the constraints as well as the objective
functional can easily be adjusted to specific application
requirements, our method provides a flexible and powerful
tool for shape optimization. We use manifold harmonics to
derive a reduced-order formulation of the optimization
problem which guarantees a smooth offset surface and speeds
up the computation independently from the input mesh
resolution without affecting the quality of the result. The
constrained optimization problem can be solved in a
numerically robust manner with commodity solvers.
Furthermore, the method allows to simultaneously optimize an
inner and an outer offset in order to increase the degrees
of freedom. We demonstrate our method in a number of
examples where we control the physical mass properties of
rigid objects for the purpose of 3d printing.",
pages = "to appear--9",
month = aug,
number = "4",
event = "ACM SIGGRAPH 2015",
journal = "ACM Transactions on Graphics (ACM SIGGRAPH 2015)",
volume = "34",
location = "Los Angeles, CA, USA",
keywords = "reduced-order models, shape optimization, computational
geometry, geometry processing, physical mass properties",
URL = "http://www.cg.tuwien.ac.at/research/publications/2015/musialski-2015-souos/",
}

